

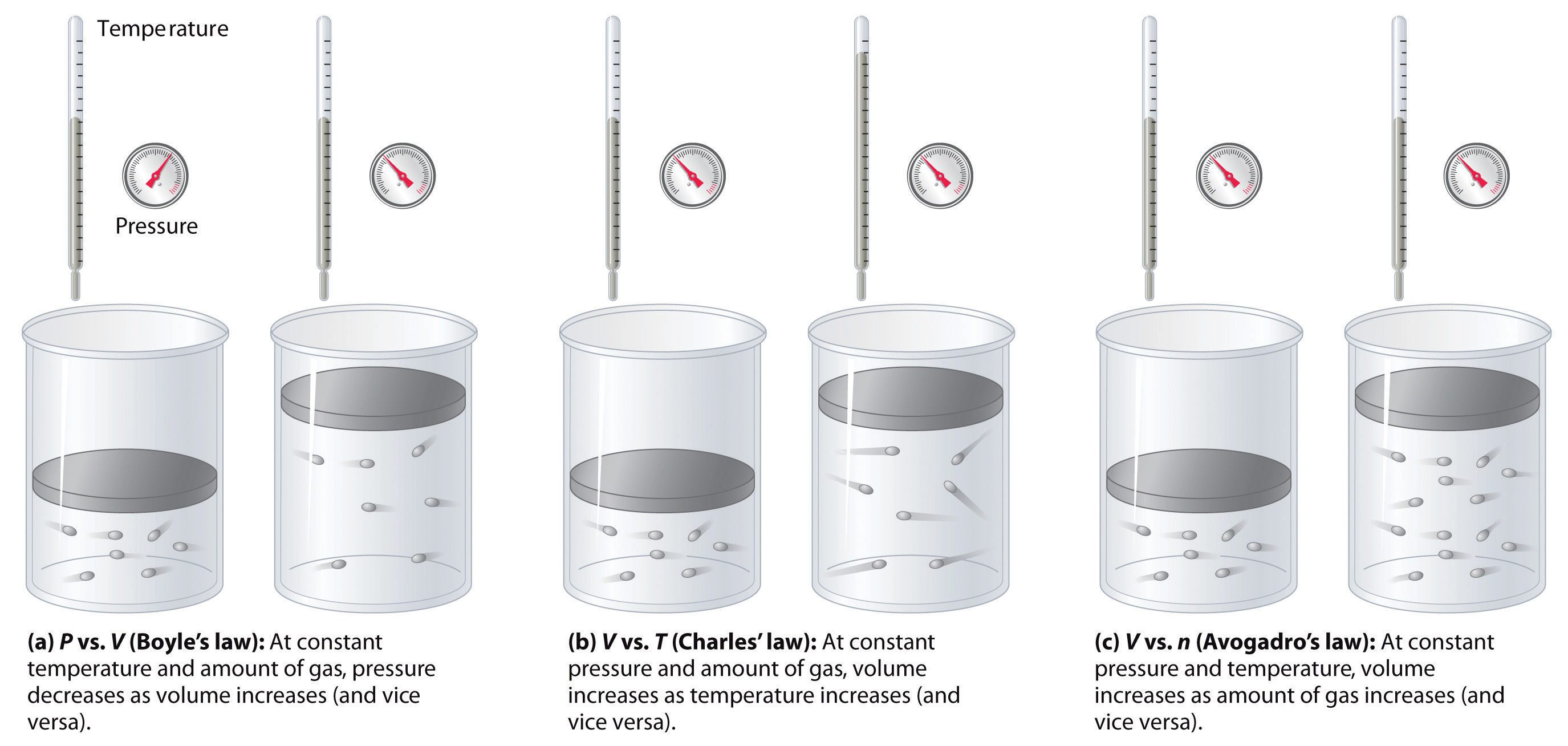
I've tried using the ideal gas law: PV=nRT but i can't seem to get where I am getting lost. The sample is then warmed to 298K during which the N2O4Ĭalculate the Volume occupied by 1.5 moles of an ideal gas at 25 degrees Celsius and a pressure of 0.80 atm. A flask of volume 5L is evacuated and 43.8 g of solid N2O4 is introduced at 77K. Having some confusion with this question.I have the answers, just not able to reach them.
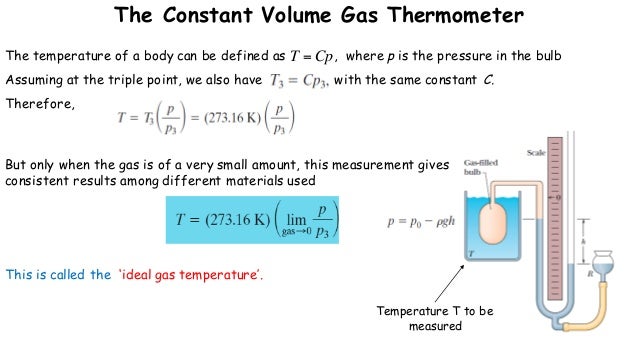
If the volume of this sample of gas is doubled and the pressure of this sample of gas is doubled, what happens to the number of moles of the gas Select one: a. An electric heater adds 52600 J of energy into the gas while the piston A sample of gas is held at constant temperature. The gas is initially at a temperature of 300 K. Here V tpis the volume of the gas at the triple point of water and V is the volume of the gas at the system temperature.If I contain 3 moles of gas in a container with a volume of 60 liters and at temperature of 400 K, what is the pressure inside the container? Ideal Gas Law: PV=nRT I know I'm given the volume, temperature, and numbers of moles ofĪ cylinder with a movable piston contains 14 moles of a monatomic ideal gas at a pressure of 2.26 × 105 Pa. Therefore , asĪ constant pressure thermometer also can be used to measure the temperature. The correct temperature of the system can be obtained only when the gas behaves like an ideal gas, and hence the value is to be calculated in limit P tp 0. This behaviour can be expected since all gases behave like ideal gas when their pressure approaches zero. When these curves are extrapolated to zero pressure, all of them yield the same intercept. If 4 moles of gas are added to a container that already holds 1 mole of gas, what happens to the pressure inside the container due to the change in the amount of gas The volume of a gas is reduced from 4 L to 0.5 L while the temperature is held constant. A plot of the temperature T cal, calculated from the expression T = 273.16 ( P/ P tp ) as a function of the pressure at the triple point, results in a curve as shown in figure 5.5. The measured pressures at the triple point as well as at the system temperature change depending on the amount of gas in the bulb.
CONSTANT PRESSURE GAS THERMOMETER MOLES SERIES
Suppose a series of measurements with different amounts of gas in the bulb are made. Where T tp is the triple point temperature of water. The temperature of the triple point of water has been assigned a value of 273.16 K. We can obtain triple point by putting water and ice in an insulated chamber and evacuating air ( which is then replaced by water vapour). Let a similar measurement be made when the gas bulb is maintained at the triple point of water, P tp. Let the pressure of the gas be read as P. This ensures that the volume of the gas is held at a constant value. According to this equation, the pressure of a gas is proportional to the number of moles of gas, if the temperature and volume are held constant. The mercury column is so adjusted that the level of mercury stands at the reference mark S. The bulb is placed in the system whose temperature is to be measured. If the gas pressure is made to approach zero, the gas behavior follows the relationįigure 5.4 shows a constant volume gas thermometer. The perfect gas temperature scale is based on the observation that the temperature of a gas at constant volume increases monotonically with pressure. The behavior of all gases approaches the ideal gas limit at sufficiently low pressure (in the limit P 0). Table 1.1: Units for pressure where C is the slope of the X-versus-T line. This equation is only an approximation to the actual behavior of the gases. Figure 1.1: The pressure gauge on the gas thermometer, the gas thermometer and the temperature bath equipment The Pascal: 1 Pa 1 N/m21atm1.01x105Pa The Torr: 1 Torr 1 mm Hg 1atm760Torr The lb/in2(psi) 1lb/in26870.8Pa1atm14.7lb/in2. Where R is the Universal Gas Constant ( R = 8.314 J/mol K). 3 Determine the pressure coefficient of an ideal gas with a gas thermometer Additional task: Measure the linear expansion of a metal tube as a function of.
Lecture 5 : State Postulate and Zeroth- Law of Thermodynamics


 0 kommentar(er)
0 kommentar(er)
